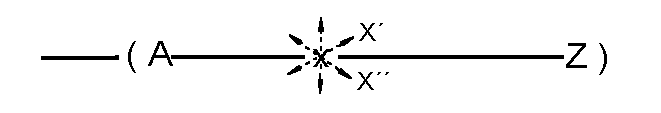| ACHILLES AND THE TORTOISE by GOTTHARD GÜNTHER |
|||||||||
| Relativity shows that nothing can move in space faster than light. The interstellar cruiser becomes possible only if we can, somehow, transcend that limitation. And one way may be simply that of asking, and finding a new answer, to the deeply fundamental question, "What do you mean by ´motion´?" | |||||||||
- part 2 of 3 - |
|||||||||
| The solution of a riddle or
of a paradox is usually something in the nature of an anticlimax. The suspension is
released, and the feeling of exciting wondermemt has passed. A famous. example is the
story of the Gordian knot. When Alexander the Great conquered Asia he found in the city of
Gordius an old chariot its yoke fastened with a cord that was tied in a complicated knot.
The cord was so artfully twisted that no one had ever been able to loosen it. Moreover,
there was an oracular Prophecy that he, who first would untie the knot, should rule Asia.
Alexander tried, but he, too, failed. So he took his sword and "untied" the knot
by cutting it in two. I heard this story first, as a small boy in school. But even then I had an uneasy feeling. This solution seemed to me rather-untidy. Hardly more than a fraud. Sure, I was given the usual interpretation of that famous incident: There are problems the intellect cannot solve and only too often Man arrives at a puzzling impasse where only daring action can find a way out. This explanation did not satisfy me at all! Who said in the first place that the Gordian knot was untyable in the precise sense of the word? Well, no one ever did. The story only tells us that many tried, but nobody succeeded. That only proves the candidates were never good enough. And look at Alexander himself! He "solved" the problem in a manner of speaking, and got his Asiatic empire. But it was the most short-lived empire in the history of Man. It fell apart the day he died. It seems his method of untying the knot was only his private solution, not valid for anybody else. The conventional solution of Zeno's paradox is just about in the same category I pointed out in Part I that the riddle of Infinity, involved in the problem of motion, seems to be insoluble. But mathematicians discovered the infinitesimal calculus, i.e. a special procedure which permitted us to abandon the material concept of actual Infinity. They replaced Infinity by the operational idea of the limit. This opened for the very first time a way to demonstrate in an exact mathematical manner what every child has already learned by countless experiences: that the fast runner always overtakes the slow runner. Practically speaking, the new infinitesimal method was of paramount importance. Modern technique and industry simply could not have been developed without the limit procedure. But hardly anything was gained as far as the precise theoretical concept of motion was concerned. It remained the mystery of old and defied all attempts to analyze it in rigid logical terms. At this point an intellectually healthy and normal person is very much tempted to say: "So what if we do not understand motion? Newton's and Leibniz's calculus permits us to use it at will. And this is all that really, counts. What else do you want?" Exactly, what else could we want? Well, what about interstellar space travel? We surely want that! But the first step toward it is to understand that all known forms of locomotion are utterly and absurdly useless, when we face the problem: how to traverse interstellar distances! Therefore, our big question is: Are there any other as yet unknown and structurally different forms of motion which are neither exemplified in our daily lives nor in the motion of the planets of a solar system in their orbits? It is absolutely impossible to answer these and related questions satisfactorily unless we have a precise rational understanding of what motion really is. That means, unless we have positively succeeded in solving the problem of Zeno ¼ instead of detouring it by eliminating its crucial element of actual Infinity. The infinitesimal calculus only demonstrated. in definite mathematical terms that this mysterious X, called motion, is possible. Thank you very much! But nobody ever doubted it. On the other hand, a hundred years of symbolic logic have spoiled our taste. We want a solution of Zeno's paradox where the problem of Infinity is not carefully eliminated, but where Infinity itself takes part in the solution and provides the explanation to the riddle of motion. It is fortunate for us that Cantor's theories suggest that there is a transfinite concept of motion, and as this Cantorian idea of changing the location of an object in Space may be the mathematical basis of all future interstellar space travel it will pay off handsomely for us to have a last look at the theory of limits, and. its logical shortcomings in relation to Cantor's arithmetic of Alephs. The theory of the differential limit only means that the quantities involved are permitted to decrease beyond any given number. There is no end to this process. But the fact that they are permitted to approach the limit of the infinitely small does not mean that they actually reach it. On the contrary, the very fact that the process is unending demands by definition that Infinity is never reached. Otherwise this endless process would have an end which would be a contradiction in itself. Consequently, every actual space-interval designated by this mathematical procedure has still a finite extension. Newton realized that already. Permit me to translate a significant statement from the Latin text of his "Tractatus de Curvata Curvarum". It says:"¼ I have intended to show that it is not necessary in the method of fluxions to introduce into geometry infinitely small figures ? /1/ This seems to be borne out by practical experience in modern experimental physics. One of the leading physicists of our time wrote only recently: "The latest development of nuclear physics suggests that there exists a ´minimum length' below which no decrease is possible " /2/. |
|||||||||
| It all boils down to the important fact that our traditional
non-Cantorian system of mathematics considers Space as having a quantized structure. Space
is made up, so to speak, of individual space-quants: tiny, discrete entities of pure
extension. The limit theory only permits us to assume these space quants to be as small as
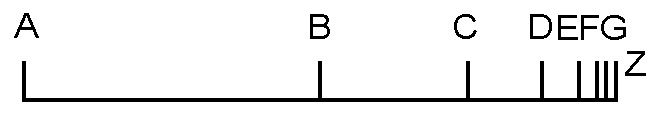
we want. If we keep that in mind, it will be possible for us to understand why we are forced to think in Zeno's paradox, that Achilles can never overtake the tortoise. In order to make comprehension easier let us again lay out our race course:
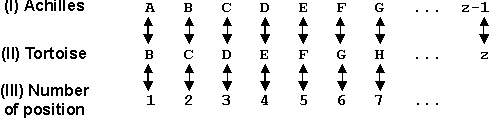
Achilles starts from A, and the tortoise begins its race at the same time from B. The finish is at Z, a point which both racers are supposed to reach at the same time; the reason being that Achilles runs twice as fast as the animal. Now, if we are required to assume that Space is quantized, then Zeno's argument will be valid and good from now to doomsday. How so? Well, let us reformulate it, and it will become evident. Zeno argues that the two racers must occupy exactly the same number of positions during their race. The following pattern of one-one relations illustrates what Zeno means:
As one can easily see, for any number of positions, n, Achilles is invariably one position behind the animal. If our hero were to catch the tortoise, he would have to occupy one more position during the same period of time. This is manifestly impossible; we have to cede that to Zeno. The intervals between the tortoise and its pursuer may progressively get smaller and smaller till they reach the order of magnitude of a space-quant. But no further decrease is possible. It follows that the number of such quants or physical (not mathematical!) points between A and Z is finite. And in the case of all finite sets a subset of a series is not numerically equivalent with the full series (cf. part 1). Achilles must stay at least one space-quant behind the tortoise. Because for a finite series of positions Z and Z-1 are not identical. Under the circumstances there seems to be no alternative left but to assume that the "smallest" segments of the line AZ have no longer any measurable length. They must be dimensionless points. But not even the summation of an infinite sequence of such points would produce a line segment of any measurable extension. Achilles starting from point one - identified with A - and moving to the successive points 2, 3, 4, 5, 6, 7, 8... would never cover any distance. Because there is no distance between the points, and the points themselves have no spatial extension. All Achilles could do with his fastest running would be to stay in A. Remember the story where Alice runs with the Red Queen? Let us see what Lewis Carroll had to say about it: "They went so fast that at last they seemed to skim through the air, hardly touching the ground with their feet, till suddenly, just as Alice was getting quite exhausted, they stopped, and she found herself sitting on the ground, breathless, and 'giddy ¼ Alice looked round her in great surprise. ´Why, I do believe we've been under this tree the whole time! Everything is just as it was!' 'Of course it is,' said the Queen. ´What would you have it?' 'Well, in our country,' said Alice, still panting a little, 'you'd generally get somewhere else - if you ran very fast for a long time as we've been doing!' 'A slow sort of country!' said the Queen. Now, here you see, it takes all the running you can do, to keep in the same place. If you want to get somewhere else, you must run at least twice as fast as that!´" It seems Achilles and Alice run in a very fast country. Their running does not take them anywhere. So far we have naively assumed that the number of points on our line segment AZ - the race course - is either finite or infinite. But we should not forget that the whole alternative "finite-infinite" belongs exclusively to the Cantorian number system of Aleph naught. It follows that our entire reasoning up to this point was based on the silent assumption that the structure of spatial extension - the continuum - can be adequately defined in terms of À 0. Anybody who states that Zeno's paradox resolves into a problem of limits makes this very assumption. The classical alternative of finite infinite was, of course, unavoidable until very recently because it was believed, that the traditional concept of the ordinary Infinite represented the upper limit for all numerical systems Cantor's discoveries have shattered this belief, and we now know - approximately seventy-five years - that the arithmetical concept of À 0 is insufficient to give us a proper picture of the structural properties of the continuum. We are aware of the fact that the continuum can only be described with the help of the non-denumerable system of real numbers. This system is not merely infinite. It is transfinite and of the order of the cardinal number c. Under these circumstances it is evident that the general problem of motion depends in its solution on the nature of the continuum. We are bound to apply to it the arithmetic of non-denumerable transfinite c. Naturally our next question should be: what is the difference between the arithmetic of denumerable and non-denumerable systems when used to measure distance in Space? An elementary drawing will help us again. Let
and
be two distances which are to be measured in terms of predetermined measuring units. And no change of the unit of measuring shall be permitted between AB and AZ. If we use denumerable numbers for our purpose, we shall find that there is a definite relation between the number of measuring units and the length of a line segment. In other words: the distance AB is shorter in terms of measuring units than the distance AZ. In order to indicate what we mean we might also use the ontological - existential - formulation: there are more space-quants between A and B than there are between A and Z. This interpretation of our measuring procedure, however, is inadmissible if we define the distances AB and AZ in terms of non-denumerable numbers. We have seen in our preceding article that the order of magnitude of all real - non-denumerable - numbers between 0 and 1 is already of the transfinite cardinal order of c. The same holds for all real numbers between 1 and 2, 2 and 3, 3 and 4¼ , or 0 and 2, 0 and 3, 0 and 4¼ In fact the same quantitative relation exists generally between 0 and n, whereby n is permitted to increase without limit. In short a line a quintillionth of a millimeter long contains as many points - as designated by real numbers - as another line stretching from Earth to the last barely visible nebula in the Universe. As soon as we use the arithmetic of non-denumerable numbers we find that there is no relation between the number of real points on a line and its length. As soon as we have reached this insight we are finally ready for a genuine solution of the problem of motion, as exemplified by the race between Achilles and the tortoise. It is impossible to solve Zeno's paradox in terms of a denumerable system of cardinal numbers. Achilles would never catch the animal ahead of him if there existed a rigid and invariant relation between our method of counting and the objective structure of Space. By "invariant relation" I mean a relation to the effect that the number of points we might count - no matter what technique of counting we might use - would always indicate the length of the measured line segment. Zeno's thesis that Achilles must occupy as many positions as the tortoise is and remains unassailable. Equally true is that he must travel a greater distance than the animal. And if the greater distance contains more real points than the smaller one then it is impossible for him to catch up. This would be the case, indeed, if the ultimate reality of our space-time continuum could be 'adequately described in terms of denumerable numbers. Zeno discovered his paradox because he used only the denumerable numbers of the system of Aleph zero. By telling his story of Achilles and the tortoise he demonstrated the inadequacy of the classical number concept. The paradoxical situation which develops between Achilles and the animal clearly demonstrates that the problem of motion in space needs for its treatment a very different concept of number. Motion is a problem of the continuum, and therefore in its general form only treatable by an arithmetical system of transfinite magnitude. Zeno, of course, could not know this. He, therefore, drew from his absolutely correct thesis, i.e. that during the race Achilles must occupy the same number of positions as his competitor', the erroneous inference that in doing so he could not travel further than the tortoise. His conclusion would have been correct only if the run from A to Z actually contained more points - real numbers - than the shorter course from A to Z. This, we know now, is not the case! Therefore the solution to Zeno's problem is: In the arithmetic of non-denumerable numbers Achilles occupies between A and Z no more and no less positions than the tortoise between B and Z. Thus it is possible for Achilles to travel a longer distance than the animal although he occupies during the race the same number of positions -as Zeno correctly pointed out - as his opponent /3/. This teaches us a fundamental lesson for all future interstellar space travel: The objective distance between two points in Space, let us say, between Earth and the Crab Nebula, can never be established by counting the absolute number of points in between. No matter how short or how long a line segment, it always has the same number of real points, and the number in question is invariably the transfinite cardinal number c. The following statement may be difficult to digest even for a willing reader, but it is true just the same: measured in the system of real numbers the distance between Earth and Crab Nebula is neither longer nor shorter than the space-interval between Earth and Moon. We naturally struggle against this revolutionary idea because we have become accustomed through thousands of years to measure distances exclusively by dint of the denumerable order of cardinal numbers. But as long as we only count denumerable numbers we cannot obtain a proper concept of either Space or Motion because both phenomena involve actual Infinity. |
|||||||||
How little we know about the basic structure of Space in general
can be deduced from the most fantastic result Georg Cantor was forced to accept when he
investigated the properties of real numbers. We learned in the preceding article that
This formula certainly looks harmless. It seems to be trivial. But it contains ontological dynamite because it means that any line - finite as well as infinite - contains as many real points as the square over it. We shall skip the demonstration of this amazing relation between a one-dimensional line and a two-dimensional plane- which, incidentally, is dome with the help of Cartesian coordinates - and proceed to the next formula
Translated into ontological terms it says no move and no less than that the number of real points of the shortest line segment is numerically equivalent to the number of all real points in an infinite three-dimensional universe. When Cantor in 1877/78 intended to publish this almost unbelievable result the editor of the mathematical periodical refused to print his article. It took the intervention of the mathematician K. Weierstrass, who had already obtained world wide recognition for himself, to get Cantor's paper on the pages of the Journal /4/. However, this is not all. We further know from our first article that
This means that all real points of any n-dimensional universe - where n is a finite number - are of the same numerical order as the number of all real points of our smallest line segment. And finally we have
In plain words, the same relation would even hold if there were a universe with an infinite number of dimensions. I am not going to discuss the far-reaching implications of the formulas (3) and (4) in this article. Formula (2) will suffice as far as our immediate problem is concerned. According to (2) it is possible to establish a one-to-one correspondence between the points of the smallest possible line segment and all spatial points of our threedimensional universe. Our line segment has "as many" points as the entire metagalactic Space. Let us put it differently: The non-denumerable order of transfinite c makes spatial dimensions and distances disappear! It should, however, be noted that the one-dimensional mapping of all space-points can only be done discontinuously. There is no continuous one-one relation between the points of a line and the points which establish the other two dimensions. That means, the transfinite system of real numbers provides us with a picture of Space which is allowed to shrink without limits. But by doing so it retains even in the form of a line segment certain characteristics which indicate that it is potentially much more than a one-dimensional sequence. The discontinuous character, of the one-one relation between a simple line segment and Space hints at the possibility that our line may at any time - provided the necessary metrical conditions exist "explode" into a three-dimensional spatial continuum. As it is almost impossible to realize at the first reading the almost unbelievable consequences of formula (2) I shall try to outline them with a few words. First, as the shortest and the longest line segments are arbitrarily interchangeable - when measured in terms of transfinite numbers - it is metrically possible to arrive at any distance from any even point by traveling a negligible minimum length in terms of finite numbers. But the second consequence is even more fantastic. According to classical concepts of geometry one can only - traveling along a one-dimensional line - arrive at points which are located on this very line. This limitation does not exist in realms where formula (2) is valid. If a finite line segment represents transfinitely a three-dimensional continuum, then it must "contain" a transfinite number of points which are - spatially speaking - not located on the very same segment where we find them. This sounds like complete madness. But don't forget, if you had submitted the blueprints of a jet plane or a television set to Moses, Alexander the Great, or Sitting Bull these gentlemen, too, would have decided that your drawings could be nothing else but the insane products of a hopelessly diseased mind. So let us face it, formula (2) implies that any line segment "contains" points which are not located on it. Let us assume, we travel along a line XZ, and we permit the line at a certain location I to "explode" into a three-dimensional continuum-location x, if unite, contains all the points required for the "explosion"- then, instead of arriving at point X we may arrive at, point X' or neither of which is located on our line of travel. |
|||||||||
It is hardly possible to overestimate the importance of these transfinite properties of Space for theory and practice of. interstellar space-travel. We are beginning to know nowadays that our present mathematical methods do not give us an adequate picture of the dimensional Space and Time properties of our universe. They describe at best the properties of Matter in our world, but not the principles of extension per se. Therefore, they fail completely when challenged to define the basic characteristics of the four- dimensional continuum of Space and Time in which our physical existence is embedded. Zeno's paradox makes it obvious, that our conventional ideas of distances and lengths are derived from our familiar knowledge of physical bodies. They apply to bodies, indeed, and generally to all varieties of material existence which has a quantized structure, but they do not apply to a different form of existence: the existence of the continua of Space and Time. If Achilles could overtake the tortoise in our present world of Aleph naught - that is, if we could think the problem of motion by using our classical "'geometrical" concept of distance, then the same concept would equally apply to interstellar distances. It is not probable that we would ever reach the stars under these circumstances. Because the idea of a journey that would take centuries even to reach our next neighbor Proxima Centauri, is absurd. And how would galactic empires - the type Isaac Asimov has described in his Foundation novels - exist, if a message from one end of our galaxy to the other side of the rim took approximately one hundred thousand years? But interstellar travel is, theoretically speaking, an undeniable certainty because the secret of motion is that it does not happen on the basis of quantized physical conditions where distances gradually pile up to almost immeasurable orders of magnitude Everybody knows from his own practical experience that Achilles catches his animal - although the theory tells us that he cannot possibly do so. This is irrefutable proof that the quantized thinking of À 0 does not apply to the problems of space. The continuum is of transfinite order, and here our traditional ideas about extension about distance, and about dimension become, invalid, and have to be redefined. Thus the impending space age will force upon Man a revolution of thinking. I should like to quote several statements of John W. Campbell, Jr. which were contained in a letter (June 24, 1953) addressed to the present writer. We were discussing Cantor's theory of the transfinite Alephs, /5/ and our editor wrote: "To date, I feel that no satisfactory correlation of Cantor's ideas with the real universe has been published. Some of the implications of Cantor's work are most disturbing to the mind orientated entirely on the quantized thinking implicit in two-valued logic, in a digital-ordered system of thinking, and in quantized physics (¼ ) One of the things implicit in Cantor's work is that if any line contains Aleph- n points, then if we accept the proposition 'Things equal to the same thing are equal to each other', we must also accept that a line of any length is equal to a line of any other length! The concept 'greater than' as applied to line segments must then be re-examined. (¼ ) If, as Cantor's concepts imply, 'length' is a fiction derived from a limited operational method, the 'distance' between two points is purely a matter of measurement! (¼ ) It seems to me that there are many indications that the whole concept of geometry is a special case of something far more general, in which Cantor's concept of Aleph-null becomes simply the first-order unit. (¼ ) And in that system, by recognizing that distance is purely a matter of operational method (¼ ) why, the stars are as near as we wish them." Please compare these remarks with the result of our preceding. article on Achilles and the Tortoise. We learned that existence and - all existence is physical existence - can only be conceived in terms of quantized thinking, be measured with digital ordered number systems, and objectively explained in quantized physics. But we also learned that all these methods fail if we want to tackle the problem of Space. The most striking indication of this failure is the existence of Cantor's formula:
according to which the smallest line segment has "as many" real points as there are in an infinite universe with an infinite number of dimensions. This elicits, of course, the question: How small is our line segment permitted to be? There is only one logical answer: As small as we can measure it. And how small can we measure it? This time there is only one physical answer: Down to the order of magnitude of one space-quant. We are, therefore, entitled to say that one single space-quant which is, an absolute unit in terms of denumerable numbers contains as many points as any n-dimensional universe - where n is permitted to increase without limit. Let us re-formulate this most important result from a different aspect. It is implied by (4) that our technique of measuring by gradual accumulation of length-units is valid only when applied to physical states of existence. It is meaningless when applied to that which "contains" all -physical existence, i.e. to empty Space per se. The concept of distance is meaningful only with regard to Substance in its two manifestations as matter and energy. It does not signify anything with regard to the voidness of Space. Talking in strictly physical terms we may, therefore, say: Space per se does not exist. But this is by no means all there is to it. We shall learn something more by having a look at the recent history of physics. Newton still believed in an independent "physical" existence of the absolute voidness of Space per se. Famous is his experiment with a rotating pail of water. Everybody knows that if a pail rotates the water will assume a concave surface. This is the effect of a centrifugal "force" engendered by the rotation, and Newton interpreted this force as the result of motion relative to absolute or empty space. The validity of his argument was first doubted by Ernst Mach. But proof that Newton must be wrong was only obtained when Michelson and Morley performed their well-known "ether-drift" experiment and Einstein discovered its proper interpretation. If earth moves through absolute space, then the apparent velocity of light should be greater when the observer moves towards its joint of emission, and smaller when he moves away from it. According to our classical conceptions this should be so, because in the first case one has to add the velocity of the observer to the speed of light, and in the second case the velocity of the observer must be subtracted as the light has to catch up with him. But when Michelson carried out his famous experiment no such change in the relative velocity of light was observed. No matter whether the observer moved towards the source of light or away from it the velocity of light remained constant at 186284 miles per second (in vacuum). Classically speaking, this is perfectly absurd. Let me illustrate it with a trivial example of our everyday life. We shall assume, there are two cars on the highway, both equipped with faultlessly registering speedometers. The first car is driven at a speed of exactly 60 mph. And the second car at 62 mph. It stands to reason that the second driver will gradually overtake the first; and when he does so he will pull ahead with exactly 2 mph relative to the first car. But the negative result of the "ether-drift" experiment suggests that the second driver would pass the first car with a speed margin of exactly 62 mph. "But that is impossible!" you will say. "If the relative speed of the two cars at the moment of overtaking is 62 mph, and the first does 60, then the second car should have an intrinsic roadspeed of 124 mph. It is impossible that the speedometer of second car indicates 62 mph. But in case it does, it is out of the question that the velocity of the two moving objects on our highway relative to each other is 62 mph. It is then exactly 2 mph." The argument is perfectly correct. The application of the Michelson-Morley experiment to our highway situation is nonsense. Because there is a highway, and both cars have two velocities - an "absolute" one with regard to the highway, and indicated by their respective speedometer readings, and a relative one with regard to each other. And their relative motion always depends on their "absolute" velocities. It can be calculated by a simple arithmetical procedure. In the case of an overtaking you subtract the smaller speed from the greater. If it is a collision, you add the two speeds to each other. But there is "no highway in the sky!" This was Einstein's solution when he tried to reconcile the negative result of the "ether-drift" experiment with our traditional conceptions of motion. Michelson expected a positive result for his experiment because he assumed that both, the light as well as its observer, would have an absolute velocity with regard to absolute Space (ether), and in absolute Time, in addition to their relative speeds. But the experiment was negative, and Einstein concluded that there was only one explanation for its result: absolute continua have no independent physical existence. Einstein insisted that we should distinguish between Space and spatiality, and Time and temporality. Spatiality and temporality are basic properties of physical events. Absolute Space and Time, however, are mere theoretical abstractions without objective reality. It stands to reason that you cannot measure abstract concepts in terms of centimeters or seconds. On the other hand, spatial and temporal properties of physical objects or processes can be measured. And this, by the way, is the scientific criterion of real existence. Nothing can be admitted as being objectively real in our Universe unless it can be measured either directly or indirectly /6/. But empty Space and eventless Time are not measurable. Their "properties" are always the same regardless of the speed or the position of the observer who is moving through them. To put it differently: It is impossible to measure distances in absolute Space or intervals of absolute Time. As far as Time is concerned readers of science-fiction magazines are quite familiar with the relativity concept. Most of them know that, if we could travel around the whole "circumference" of the Universe in, say, a dozen years spaceship time, and we returned to Earth, billions of years would have elapsed in terrestrial time. But so far it has occurred only to a few that the distance Earth-Andromeda Nebula may be about two million light-years, measured in terms of terrestrial physics, but hardly anything, measured from a spaceship under proper space-travel conditions. This is at least theoretically possible, because distances in absolute space are nonexistent. Even more, it is meaningless to combine the idea of distance with that of empty Space because relative to absolute Space the shortest imaginable and the longest imaginable distance are numerically equivalent. This result was previously implied by Cantor's formula:
How about space travel now? I am afraid I shall have to postpone my answer to that fascinating problem till the final article, because one important link between the different parts of our puzzle of motion is still missing. We have established that neither Time nor Space are absolute data of Reality. We are beginning to realize that the technique of interstellar and even intergalactic space travel will probably not be hampered by the consideration of millions of years and billions of parsecs. But the physical reality of our Universe is obviously a product of three basic components: Space, Time and - Matter. So far we have only heard about the relativity of spatial and temporal characteristics of our Universe, but nothing about its material component. Is there also a relativity of Matter, or is material existence the absolute and irreducible core of Reality? My third and last article intends to show that Matter is as relative as Space and Time. And it is just this reciprocal relativity of Space, Time, and Matter which will enable us to understand that interstellar and intergalactic travel is not the product of the feverish fantasy of some science-fiction writers, but a theoretically well grounded implication of modern physical science. Footnotes: /1/ In the orginal text:"¼
volui ostendere quod in methodo fluxionum non opus sit figuras infinite parvas on
geometriam inducere". Incidentally, "method of fluxions" is Newton's
original name for the differential calculus.
/2/ Cf. C. F. von Weizäcker, Zum Weltbild der
Physik, 4th ed. Zürich 1949, p.145.
/3/ A note to
mathematicians: yes I know that Cantor´s "positive theory of Infinite" provides
a solutions to Zeno´s problem only if we do not identify mathematical
"existence" with construction. However, if we do - as the revolutionary school
of mathematical inuitionists (Kronecker, Brouwer) insits we should - Zeno´s problem is
matbematically speaking still unsolved. But Kronnecker's "revolution" would
banish all but the positive integers from mathematics. This seems rather larger order!
/4/ Cf. Journal f. Math.
vol.84, pp.242-252, 1878.
/5/ Cf. Astounding,
July'1954, pp. 76-88
/6/ Cf. P. W. Bridgman,
The Logic of Modern Physics. New York 1927.
|
|||||||||
| Günther Web | |||||||||
vordenker Contact: webmaster@vordenker.de
|
|||||||||